
| Physics I – 12 CFU | |
| DidatticaWeb | |
| richetta@uniroma2.it | |
| +39 06 72597197 | |
| Office Location | Bldg. Ingegneria Industriale room 3135/9 |



| Physics I – 12 CFU | |
| DidatticaWeb | |
| richetta@uniroma2.it | |
| +39 06 72597197 | |
| Office Location | Bldg. Ingegneria Industriale room 3135/9 |

| 1 YEAR | 2 semester | 12 CREDITS |
| Maria Richetta | 2019-20 2020-21 didatticaweb |
| Emmanuele Peluso – emmanuele.peluso@uniroma2.it |
2021-22 to 2023-24 |
| didatticaweb Code: 8037948 SSD: FIS/01 |
OBJECTIVES
LEARNING OUTCOMES:
Students will improve their knowledge under three main aspects: a) the comprehension and the related capability to articulate the arguments discussed throughout the course, using the proper scientific vocabulary and style; b) the methodological attitude toward complex problems, more specifically, to decompose a physical phenomenon into simpler elements to reach efficaciously a correct explanation; c) an elastic, fluid and reactive way of thinking, based on an inquisitive and hungry developed attitude toward new challenges.
KNOWLEDGE AND UNDERSTANDING:
Knowledge of the main arguments related to classical mechanics, gravitation, mechanical waves, elastic properties of solids and thermodynamics. Identification of the physical quantities and of the conservation principles needed to explain the main physical phenomena behind the above reported topics. Understanding scientific texts related to the course, and the acquisition of proper vocabulary and style.
APPLYING KNOWLEDGE AND UNDERSTANDING:
Capability to achieve the correct solution of complex classical mechanical, gravitation, mechanical waves, elastic properties of solids and thermodynamics problems, through an effective and methodologically correct understanding and application of the principles governing the physical phenomena described. Capability to apply the acquired scientific way of thinking to new problems by comprehending, analysing and also modelling autonomously.
MAKING JUDGEMENTS:
Students are supported to improve their critical way of thinking and to develop an independent judgment able to pose, refine and elaborate scientific questions. The purpose is to pave the way to a free and active research spirit based on a questioning elastic and hungry mind.
COMMUNICATION SKILLS:
The students are expected to master, through familiarity with the arguments studied and through a developed practice refined in the framework of the course, a specific focus, style and proper scientific vocabulary.
LEARNING SKILLS:
Capability to understand, analyse, and model a physical phenomenon crossing different principles learnt during the course. Considering problems related to the topics of the course, students are expected to solve them and articulate the reasons behind the main assumptions followed. An inquisitive attitude, a proper scientific way of reasoning and communicative skills are therefore expected to be acquired during the course.
SYLLABUS
Mechanics (main arguments considered):
• Measurements and fundamental quantities; coordinate systems; elements of vector calculus;
• Relative motion and Galilean transformation; Inertial and non-inertial frames of reference; Fictitious forces;
• The point mass concept; kinematics; from rectilinear to general curvilinear motion on a plane; dynamics; the principle of conservation of momentum; Newton’s laws; the concept of force as interaction; angular momentum; torque; impulse; energy and work: conservative and dissipative forces; conservation principles; discussion of potential energy curves.
• From the point-mass to systems of particles: external and internal forces; Center of Mass (CM): definition, calculus and dynamics; angular momentum and torque for systems; the CM and laboratory frame of reference; König theorem part I and part II; energy of a system; reduced mass;
• Collisions: generality, impact parameter; elastic, partially inelastic and perfectly inelastic collision;
• From systems to the rigid body; moment of inertia: main properties and relationships; statics and dynamics; work on a rigid body; kinetic energy of a rigid body; pure rolling and sliding; rolling friction; systems with variable mass;
• Oscillatory motion: simple harmonic motion; energy of simple harmonic motion; superposition of simple harmonic motions; coupled oscillators; damped oscillations; forced oscillations;
Elastic properties of solids (main arguments considered):
• Young, Shear and Bulk moduli; elastic and plastic deformations; elastic hysteresis.
Gravitation (main arguments considered):
• Kepler’ s laws; Cavendish experiment; Newton’s law of universal gravitation; gravitational potential; a reduced mass approach; bounded and unbounded orbits; escape velocity; geostationary orbit;
Mechanical waves (main arguments considered):
• generality; transverse and longitudinal waves: velocity and equation of the waves; sound waves; intensity of waves; interference; Doppler effect; supersonic waves;
Fluids (main arguments considered):
• Introduction to fluids; mechanical actions on fluids; statics of fluids; Torricelli’s experiment; Archimede’ s principle; Pascal’s principle; fluid and conservative forces; fluids in non-inertial frames of reference; fluids dynamics: Lagrangian and Eulerian approaches; stationary motions; volumetric flow rate; Bernoulli’ s equation; Venturi’ s effect; considerations on real fluids: laminar and turbulent flows; viscosity; Reynolds’ number; considerations on drag forces in fluids;
Thermodynamics (main arguments considered):
• Main concepts of thermodynamics; zeroth principle of thermodynamics; the first principle of thermodynamics; internal energy, heat and work; Ideal gases and ideal gas law; P-V diagram; molar specific heats; heat capacity; main processes in thermodynamics; real gases; Wan der Waals equation; phase transitions; second principle of thermodynamics; Carnot cycle; Carnot theorem; Clausius theorem; entropy; thermodynamic Universe; Enthalpy, Helmholtz free energy, Gibbs free energy; Dalton’ s principle.
Texts
Students can use the book that best suits them. However, the references cited below are the ones considered during the course:
1. “Fundamental University Physics Volume 1: Mechanics”, by Alonso & Finn
2. “Fundamentals of Physics”, by Halliday & Resnick
3. “Physics for Scientists and Engineers”, by Serway & Jewett
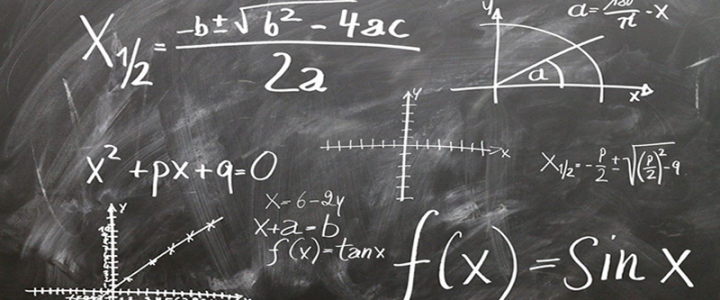
| 1 YEAR | 2 semester | 9 CFU |
| Prof. Paolo Salvatore | 2019-20 |
| SALVATORE PAOLO – LHOTKA CHRISTOPH HEINRICH |
2020-21 |
| Francesca Tovena – tovena@mat.uniroma2.it Andrea Santi |
since 2021-22 |
|
Andrea Santi (6 cfu)- santi@mat.uniroma2.it |
since 2023-24 |
|
Emmanuele PELUSO |
2024-25 |
| Code: 8037949 SSD: MAT/03 |
OBJECTIVES
LEARNING OUTCOMES: The course provides an introduction to linear algebra and euclidean geoemetry.
KNOWLEDGE AND UNDERSTANDING: The student will learn to solve simple geometric and algebraic problems using the tools provided by the course.
APPLYING KNOWLEDGE AND UNDERSTANDING: Ability to apply knowledge and understanding to concrete problems.
MAKING JUDGEMENTS: The student will learn how to interpret the data of an algebraic or geometric problem without following standard schemes.
COMMUNICATION SKILLS: The student will show, esapecially during the oral exam, her/his ability to describe the logical process that yields the theorems studied in the course.
LEARNING SKILLS: The student will learn to understand the exercises of the written exams, and to develop a method to solve them.
COURSE SYLLABUS
Linear equations and linear systems. Solutions. Consistency of a system. Basic and free variables. Matrix of coefficients. Augmented matrix. Row reduction to echelon matrix. Exercises on linear systems. Numerical vectors. Addition and multiplication by scalars. Linear combinations. Linear systems and vectors. Linearly independent vectors. Finding subsets of linearly independent vectors. Linear systems in matrix form. Exercises on linear systems in vector form. Canonical basis. Linear space. Basis and coordinates of vectors. Steinitz lemma. Dimension of linear spaces. Rank of a matrix. Linear spaces of rows and columns of a matrix. Null space of a matrix. Matrix transformations. Injectivity, surjectivity and rank. Linear transformations and matrices. Multiplication and addition of matrices and their linear transformations. Invertible matrices. Computing the inverse via row reduction Change of coordinates and matrices Vector (linear) spaces. Examples of polynomials and matrices. Linear subspaces. Intersection of linear subspaces. Sum of linear subspaces. Grassmann formula. Basis for intersections and sums of linear spaces. Determinants: definition, properties, computation. Computation of the rank using determinants. Computation of the inverse matrix using determinants. Determinant of a product. Cramer’s formula. Linear transformation between vector spaces. Image and kernel. Matrix of a linear transformation with respect to basis of the domain and of the range. Lines in the plane and in 3-dim. space. Planes in the 3-dim. space. Cartesian and parametric equations. Lines through 2 points. Plane through 3 non collinear points. Relative position of two planes. Relative position of two lines in 3-dimensional space. Inner product. Norm. Distances. Orthogonal vectors, lines, planes. Angles. Cross product in 3-dim. space. Mixed product. Area of parallelogram. Volume of parallelepiped. Eigenvalues and eigenvectors. Characteristic polynomial. Algebraic and geometric multiplicities. Diagonalization of endomorphisms and matrices. Orthogonal subspaces, orthonormal basis, orthogonal matrices.
Gram-Schmidt orthonormalization. Formula for the orthogonal projection. Matrix of orthogonal projections. Spectral theorem for symmetric matrices. Quadratic forms and their classification.Conic curves: classification Rotations and translations that put a conic in normal form.

| 1 YEAR | 2 semester | 9 CFU |
| Flavio Lombardi | 2018-19 |
| Enrico Simeoli | 2019-20 |
|
Walter Liguori |
since 2020-21 |
| Gianluca ROSSI | 2023-24 |
|
Cesare ROSETI Mauro DE SANCTIS Tommaso ROSSI |
2024-25 |
| Code: 8037947 SSD: ING-INF/05 |
OBJECTIVES
LEARNING OUTCOMES:
The course aims to provide students with knowledge and skills for effectively using computer methodologies and tools in the engineering field, especially for developing algorithms.
KNOWLEDGE AND UNDERSTANDING:
Acquire knowledge of the internals of computer architectures.
Acquire knowledge of data structures and algorithms.
Acquire knowledge of the principles of programming languages, including the object-oriented paradigm and tools and techniques for software development.
APPLYING KNOWLEDGE AND UNDERSTANDING:
Acquire the ability to analyze problems and design and implement software artifacts addressing them.
Acquire the capability of a group working on software development and documentation.
MAKING JUDGEMENTS:
Being able to choose appropriate languages and tools for software development.
Being able to evaluate the correctness and efficiency of a software implementation.
COMMUNICATION SKILLS:
Be able to describe and document software artifacts correctly and effectively.
LEARNING SKILLS:
Using the technical documentation and the reference manuals of systems, products and languages effectively.
COURSE SYLLABUS
“Introduction to the computational method. Von Neumann architecture. Programming languages: assembler, compiled, and interpreted languages. Basic concepts of programming languages: variables; data types and assignment; control structures (loops, conditional selection); basic data structures; input and output. Functions and recursion. Searching and sorting algorithms. Computational complexity. Dynamic data structures: array; linked lists; trees; hash tables. Object-oriented programming.
The programming languages taught are Python and C.”